Physics 213 Final 14 Points Each December 16, 2004

Instructor: K. W. Nicholson Please circle your answers.

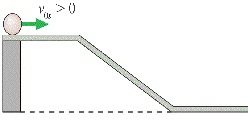
1. Draw the x vs t , v vs t, and a vs t curves for the ball’s motion until it rolls off the right edge of the track.

2. A 200 kg weather rocket is loaded with 100 kg of fuel and fired straight up. It accelerates at 30 m/s² for 20 seconds before running out of fuel.

2 a. At what instant after liftoff does it reach it’s maximum altitude? (Use 10 m/s² for the acceleration of gravity.)

2 b. Draw a velocity vs time graph for this rocket from liftoff until it reaches its maximum altitude, and use this graph to obtain the rocket’s maximum altitude.

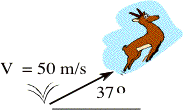
3. Gi-Gi, the famous Daredevil Gazelle plans to emulate a home-run at the opening game of the season for the Braves. The back fence is 150 m from home plate and 30 m high. If Gi-Gi leaves the ground with a velocity of 50 m/s at an angle of 37^o above the horizontal, does she make a home-run ? If not, at what height does she hit the wall?

1. Draw a picture and impose a reference frame on this picture. 3 pt

2. Draw motion diagrams in both vertical and horizontal directions. 2 pt

3. Mark on picture points A and B. 1 pt

4. Write down in neat column form, values for x, v_x , a_x,, y, v_y , a_y and t at each point. If you do not know the value, write down the equation for that value. 4 pts

5. Finally, for 4 points, solve the problem!

(The following problem was taken from the infamous Saga of Hank & Sally . For those of you that weren't here for these exciting episodes, the bag of goodies has a mass of 50 kg.)

4. The dazed, but unharmed, orangutan finally recovers her composure, and leaps to her feet. Unlike the bear, she is unhampered by the bag, she quickly catches up and dives onto the bag. The added 30 kg mass of the orangutan increases the friction force which slows the bear, (still applying a 60N force to the rope) down at a rate of 2 m/s².

Draw a FBD of the bag.

Is the bag-orangutan mass in equilibrium?

Sum the forces acting on the bag-orangutan mass.

Find the net friction force acting on the bag-orangutan mass.

5. The maximum speed an automobile can travel around a curve of radius 250 m on a flat highway without skidding is 40 m/s. What is the coefficient of static friction between the roadway and the tires? (you must draw a FBD to get any credit.)

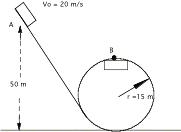
6. What is the velocity of the 50 kg block sliding down the loop - the - loop ramp at B if the track is friction free.

7. Determine the rotational mass of the system of masses in the figure if the system is pivoted about an axis perpendicular to the plane containing the masses and thru one of the corner masses.

8. Karol is skating along and throws the 30 kg ball to Karl with a velocity of 25 m/s relative to the earth. The impulse of this effort slows her to a speed of 20 m/s. What was Karol's velocity before she threw the ball ?

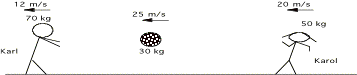
9. Fido the fat (200 kg) is sitting on a stationary merry-go-round. When Fido begins galloping clockwise around the merry-go-round a distance of 2 m from the center at a speed of 4 m/s, the merry-go-round rotates in the opposite direction 2 rad/s, what must be it’s moment of inertia?
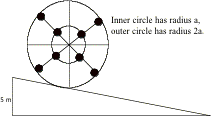
10. We have here a massless hoop system, with 8 masses, m, attached as shown. It begins at rest from the top of the incline that is 5 m high, how fast is it going when it reaches the bottom?

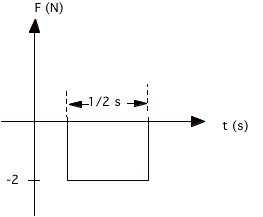
11. 5. A 200 g canary is flying east at a constant 1 m/s when she experiences a sudden force due to a gust of wind. The impulse delivered to the terrified bird is indicated in the following F vs t graph. What are the canary's speed and direction after the gust ends?

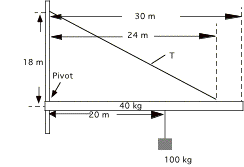
12. Find the tension T in the rope. (FBD is essential for credit.)

For these last two problems, you get half credit if you can just write down a good plan.

13. The 30 kg ball attached to a 2 m rope is released from the position indicated. Upon impact with the box, a special mechanism releases the ball from the cable on impact. The ball sticks to the box and the two slide forward 5 m as indicated. What is the coefficient of friction between the surface and the box ?

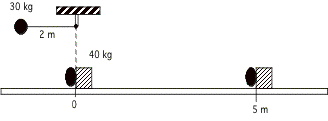
14. You have been hired to design a spring launched roller coaster that will carry two passengers per car. The car goes ujp a 10 m high hill, then decends 15 m to the track's lowest point. You've determined that the spring can be compressed a maximum of 2 m and that a loaded car will have a maximum mass of 400 kg. For safety reasons, the spring should be 10% larger than the minimum needed for the car to just make it over the top.

a. What spring constant should you specify?

b. What is the maximum speed of a 350 kg car if the spring is compressed the full amount?